Acoustics of Baseball Bats
Daniel A. Russell
Science & Mathematics Department, Kettering University, Flint, MI 48504-4898
All images and text are ©2000-2003 Daniel A. Russell
Vibrational Bending Modes of a Baseball Bat
| Mode shapes for 30-inch Little League wood bat
Fundamental bending mode (215 Hz)
Second bending mode (670 Hz)
Third bending mode (1252 Hz)
|
The animations at left show the first three bending modes of a freely supported baseball bat. The handle end of the bat is at the right, and the barrel end is at the left. The numbers on the axis represent inches (this data is for a 30 inch Little League wood baseball bat). The animations are experimental results from an measurement called modal analysis, from which the relative motions of every point on the bat may be determined compared to every other point. The amplitude of the vibration is greatly exaggerated for clarity. When excited by an impact force, such as a baseball striking the bat, all of these modes, (as well as some additional higher frequency modes) are excited and the bat vibrates. These vibrational modes can be heard quite clearly by holding the bat between finger and thumb about 5-6 inches from the barrel end and striking the bat end with a hard object. Holding the baseball bat at the handle reduces the amplitude of vibration considerably and causes the vibration to decay very quickly, but does not significantly affect the frequencies or the mode shapes of the vibration.
The fundamental bending mode has two nodes, or positions of zero displacement). One is about 6-1/2 inches from the barrel end close to the sweet spot of the bat. The other at about 24 inches from the barrel end (6 inches from the handle) at approximately the location of a right-handed hitter's right hand.
The second bending mode has three nodes, about 4.5 inches from the barrel end, a second near the middle of the bat, and the third at about the location of a right-handed hitter's left hand.
 These animations were obtained from a modal analysis experiment. The bat was suspended at each end with rubber bands. The bat was tapped with a special force hammer at each of 30 points along the bat's length. An accelerometer was fixed to the barrel end of the bat, and a measurement of the ratio acceleration/force (called a Frequency Response Function) was recorded for each of the 30 locations. A modal analysis software package (STAR Modal 5.2) was used to curve fit the FRFs so as to determine mode shapes, frequencies and damping constants. The animations at left were obtained for one of the wood bats in our laboratory.
|
Bending Modes and the Sweet Spot
There are many definitions for what constitutes the "Sweet Spot" on a baseball bat. From a player's viewpoint, the sweet spot is the place on the bat barrel where the contact between bat and ball results in the best hit - the ball leaves the bat with the greatest speed and the player's hands feel very little vibration from the impact.
Most often in the published research the sweet spot is usually refered to as either the center of percussion (COP) or the node of the first bending mode.[1] In my study of baseball bats I prefer to follow the convention used by Rod Cross[2] who defines the sweet zone as the region located between the nodes of the first and second modes of vibration (between about 4-7 inches from the barrel end of a 30-inch Little League bat). Since the vibrational motion of the bat is very small in this region, an impact in this region will result in very little vibration of the bat (no stinging a player's hands) and a very solid hit will result with maximum energy being given to the ball. An impact to the outside (towards the barrel end) or inside (towards the handle) of this zone will result in a much more significant vibration of the bat, often felt as a painful sting. And the ball will not travel as far because some of the energy is now being stored (or dissipated) in the bat's vibration.
Howard Brody[3] has convincingly shown that gripping the bat with the hands adds a significant amount of damping, causing the bat's natural vibrations to decay very quickly. However, gripping the bat with the hands has very little effect on the frequencies or the mode shapes. Thus, suspending the bat from rubberbands, as I do in our acoustics lab, to obtain a free-free boundary condition is a legitimate approach to studying a bat's behavior.
Wood versus Aluminum Bats and the Sweet Spot
Almost every bat on the market (especially newer aluminum bats) is claimed to have a "wider" sweet spot. [DISCLAIMER: quite often such claims are effective marketing ploys which are very difficult, if not impossible, to back up with scientific or technical evidence] Usually what such a claim means is that a certain bat is more forgiving for impacts outside of the sweet zone. Newer technologies such as triple walled barrels, nitrogen gas bladders, piezoelectric active damping control devices, rubber hand grips, rubber end plugs,etc., help to minimize the resulting vibration of an aluminum bat.[4-6]
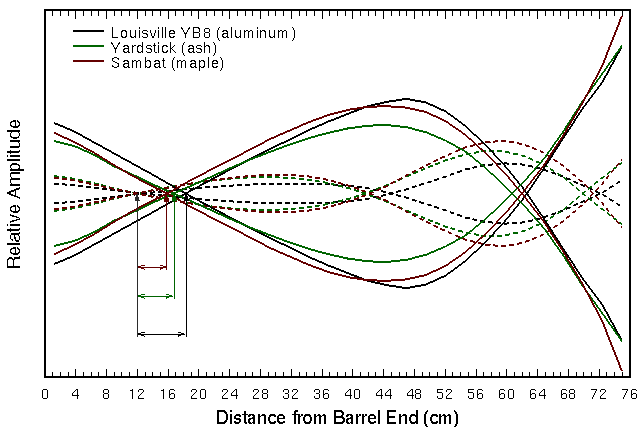
The figure below compares sweet zones for three Little League (30-inch) bats: two wood bats (ash and maple) and a single-walled aluminum bat (with no fancy technological improvements). The sweet zone (distance between nodes for modes 1 and 2) is wider for the aluminum bat than it is for the two wood bats. So, by this definition of the sweet zone, the aluminum bat has a wider sweet spot. However, the natural damping in the aluminum bat is so small that when a freely-supported bat is struck an impact at the barrel, the aluminum bat continues vibrating more than 10-times as long as a wood bat. In addition, the vibration amplitude felt by the hands is much higher for a single-walled aluminum bat than it is for a wood bat. So, while the sweet zone is wider, the resulting vibration for impacts outside of this zone is actually worse for this aluminum bat. Older aluminum bats (ie., those made in the late 70's and early 80's) actually sting worse than wood bats. The rubber handle wraps and the recent technological modifications to aluminum bats reduce the vibration so that modern aluminum bats now sting less than most wood bats.
References
[1] H. Brody, "The sweet spot of a baseball bat," American Journal of Physics, 54(7), 640-643 (1986)
[2] R. Cross, "The sweet spot of a baseball bat," American Journal of Physics, 66(9), 771-779 (1998)
[3] H. Brody, "Models of baseball bats," American Journal of Physics, 58(8), 756-758 (1990)
[4] J. Braham, "Keep your eye on the bat," Machine Design, 69(13), 56-66 (July 10, 1997)
[5] "Batter up! Piezo dampers take the sting out of swing," Machine Design, 70(15), 46-47 (August 20, 1998)
[6] J. R. Fricke, "Lodengraf Damping - An Advanced Vibration Damping Technology," Sound and Vibration, 34(7) 22-27 (2000)
Back to Acoustics of Baseball Bats